Photo by powerbooktrance.
Paraphrased from a homeschool math discussion forum:
“Help me teach fractions! My son can do long subtraction problems that involve borrowing, and he can handle basic fraction math, but problems like
give him a brain freeze. To me, this is an easy problem, but he can’t grasp the concept of borrowing from the whole number. It is even worse when the math book moves on to
.”
Several homeschooling parents replied to this question, offering advice about various fraction manipulatives that might be used to demonstrate the concept. I am not sure that manipulatives are needed or helpful in this case. The boy seems to have the basic concept of subtraction down, but he gets flustered and is unsure of what to do in the more complicated mixed-number problems.
The mother says, “To me, this is an easy problem” — and that itself is one source of trouble. Too often, we adults (homeschoolers and classroom teachers alike) don’t appreciate how very complicated an operation we are asking our students to perform. A mixed-number calculation like this is an intricate dance that can seem overwhelming to a beginner.
I will go through the calculation one bite at a time, so you can see just how much a student must remember. As you read through the steps, pay attention to your own emotional reaction. Are you starting to feel a bit of brain freeze, too?
Afterward, we’ll discuss how to make the problem simpler…
7 Steps to Subtract Mixed Numbers
Step 1. Ignore the whole numbers for a moment, and focus on the fraction parts.
Step 2. Convert both fractions to a common denominator. That in itself involves several steps:
- Look at the two denominators. Can you see a super-easy common denominator? For example, is one of the numbers a multiple of the other, or are they both divisors of some common number like 12?
- If not, then realize that the Least Common Denominator is an irrelevant sidetrack and don’t worry about it.
- Instead use the Easiest Common Denominator: Multiply the denominators of your fractions. It always works.
- Multiply the numerators of your fractions by the same amount to create equivalent fractions.
- Cross out the original fractions and write your new fractions next to them.
Step 3. Compare the fractions. Notice that the one being subtracted (which is called the subtrahend) is larger than the one you are taking it away from (the minuend). Panic. Take a deep breath. Calm down and go on to…
Step 4. Borrow/rename from the whole number part of the minuend to make a bigger fraction. This involves several more steps:
- Subtract 1 from the whole number, crossing it out and writing your new number above.
- Mentally convert the “borrowed” 1 to an improper fraction with the common denominator.
- Mentally add that converted fraction to the fraction part of the minuend.
- Cross out the fraction part of your minuend (yes, again!) and write your newer value beside it.
Step 5. Subtract the fraction part of the subtrahend from the fraction part of the minuend. Write this answer down below.
Step 6. Check to make sure the fraction part of your answer is in lowest terms. Whew! This time it is.
Step 7. Now look at the whole number part of the calculation and follow the standard rules for whole number subtraction…
I can see how a student might get confused, can’t you? And transferring the problem to manipulatives will only add steps, making it even more complicated!
Photo by gotplaid?
Make It Simpler, Part 1
I would like to try a different approach. Sometimes, taking the long way around a problem actually leads to a shorter solution. As an added bonus, the round-about method may help a student make sense out of the calculation, rather than just trying to memorize and follow a complex recipe of steps.
Here is the basic principle:
Whenever you get stuck on a problem, think about anything you can do to make it simpler.
With that in mind, let’s look again at our mixed-number calculation:
How can we make it simpler? Ignore the fractions for now. If it was 10 – 2, that would be easy:
Now put the fractions back where they were:
- Can you see that this calculation will have exactly the same answer as the problem before?
This is a key principle of working with arithmetic — do whatever you can to make your calculation simpler, without changing the basic problem. Most of the first year of algebra is spent learning how to simplify equations in this way.
Make It Simpler, Part 2
Okay, now what else can we do? If we had a really short problem like 
Now we have to put that left-over 
![8 \frac{1}{4} - \frac{3}{7} = 7 \frac{1}{4} + [ 1 - \frac{3}{7} ] = 7 \frac{1}{4} + \frac{4}{7} = ?](https://s0.wp.com/latex.php?latex=8+%5Cfrac%7B1%7D%7B4%7D++-++%5Cfrac%7B3%7D%7B7%7D++%3D++7+%5Cfrac%7B1%7D%7B4%7D++%2B+%5B+1++-++%5Cfrac%7B3%7D%7B7%7D+++%5D++%3D+7+%5Cfrac%7B1%7D%7B4%7D++%2B++%5Cfrac%7B4%7D%7B7%7D++%3D+%3F+++&bg=ffffff&fg=303030&s=3&c=20201002)
So far, we have made the problem simpler (only one mixed number instead of two), and we have taken away everything we were supposed to subtract. Our last step is a relatively straight-forward addition problem, putting the two fraction pieces together.
At this point, we are forced to find a common denominator — but at least we got the hard subtraction work out of the way first!
Show Your Work
How would this work in the “real life” of math homework? Start by crossing out the 10 – 2 and writing in the 8. It is easy to figure 8 – 1 = 7 mentally. I think most students can do 
The main thing students would need to write down would be that last line of addition:
Denise Gaskins' Let's Play Math 
Would converting them both to improper fractions with a common denominator help?
If basic fraction math isn’t a problem, then rearranging the terms might help, too.
Which is basically exactly what you’ve described, dealing with the whole number part separately from the fraction part. This is just another way of looking at it.
@Jackie—
I did offer the improper fraction suggestion to the forum poster, in addition to the method above. But I doubt she uses that idea. I don’t think many students want to deal with fractions involving such big numbers.
@Roger—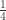
separated from the 
. Your method will still require “borrowing” from the whole number, converting the borrowed 1 to 28ths, and then doing the subtraction.
What you have described is very similar to my suggestion. The biggest difference is that I keep the
By ignoring the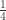
until the end, I am able to use the much easier calculation 
plus an addition problem. Most of my students strongly prefer addition over subtraction.
When I taught this to 7th graders, I used making change as an example.
First we would all agree that a quarter is 1/4 of a dollar, a dime is 1/10, and a nickel is 1/20. Then the example went like this:
“Suppose you have 3 dollar bills and 2 quarters in your pocket, and I bump into you outside the grocery store and ask if I can borrow 5 quarters — I need quarters, a dollar bill won’t help. So you go in the store and get the clerk to change one of your bills into quarters. How many quarters will she give you?”
While I’m talking them through it, I show them on the board how I’m “borrowing” from the dollars’ column and adding 4 to the fractions (quarters).
We do the same thing with nickels and dimes, and it always seemed to help a lot. It gave them something concrete to hang on to (a real-life example of a time when “borrowing” worked differently), without adding a manipulative for them to think about.
Great idea! From reading the discussion, this student seems to think that “Whole numbers are whole, and fractions are parts, and never the twain shall meet.” If he could get his mind around the analogy of making change, everything would probably come clear for him.
Denise,
It’s what I do, and when asked, how I help little kids.
There is a nice focus maintained throughout on the “big picture” – I mean, look how you never get lost in details to such an extent that we forget about the 10 – 2 part.
Very nice.
Jonathan
One more idea:
Subtract whole numbers separately: 10 – 2 = 8
Subtract the fractions separately:
1/4 – 3/7 = 7/28 – 12/28 = -5/28
It’s negative but all that means is you need to take it away from the other result you got… the 8.
All in all you have 8 and -5/28 and so the total is
8 – 5/28 = 7 23/28
Thank you, Jonathan.
Maria, I have used that idea with whole number subtraction, for a student who struggled with borrowing. He enjoyed playing with an “advanced” topic like negative numbers. I don’t think I’d want to try it on a student who was struggling with fractions, however — sounds like a recipe for brain overload.
On the other hand, I can think of one student in my elementary math club who would probably enjoy doing all his homework that way…
The way I teach my students who have trouble with this concept is what we call “consumation of differences”.
Instead of subtracting the fraction, you add to the number being subtracted, whatever fractional part needs to make it a whole number. So for your example of 10 1/4 – 2 3/7, you would take 4/7 and add it to create a whole number of 3, but to keep the problem balanced, you also add it to 10 1/4. (this also helps them with their continuity between equations and fractions)
By creating common denominators to add 10 1/4 and now 4/7, they end up with 10 7/28 + 16/28, which gives them 10 23/28 to work with, but now they are only subtracting 3 as a whole number instead of 2 3/7. So as a final step they are only subtracting the whole numbers instead of borrowing from the whole, plus working with adding to the numerator AND changing common denominators. It works really well!
I like this method! It is shorter than mine by one subtraction step — and as I said, my students have always hated subtraction.
This is also a good mental math trick for whole number subtraction problems: Add to both numbers whatever it takes to make the subtraction simpler. Consider the example…
Adding 13 to both numbers changes this to…
so can you give me an answer for this one please post it here or email it to me i got this off a web site
2 and 5/2 minus 2 and 3/2 what is the answer
its mixed numbers by the way i just dont know how to put up mixed numbers on here
Is this the calculation you mean?
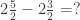
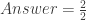
First, look at the whole parts. You have 2 whole things, and then you take away 2, so there are no whole parts left. Next, look at the cut pieces (fractions). Since they are all the same size (halves), you do not have to worry about the denominators. You have 5 pieces, and then you take away 3 of them, which means you have 2 halves left.
[Which, of course, is the same as 1 whole thing.]
what is the answer to 6 – 1 3/8? and how do you get it?
It may help to think of pizzas, Caitlin. The fractions might really represent anything, but pizzas make an easy-to-imagine model to help you figure the problem out.
. How much would you have left? Well, first you would eat a whole pizza, which would leave 5 pizzas left. Then, you take some Tums and come back for more pizza. You eat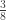 
of the next pizza. What is left after that? In addition to a horrible stomach ache, you would have 4 whole pizzas left and part of the 5th pizza.
Imagine starting with a pizza, and then eating
To make it precise: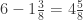
.
subtracting mixed number from mixed number and finding the least common denominator.
77 and 65/90
–
58 and40/75.
Let’s start with the fractions this time. For a common denominator, you need to make a multiple of 90 and 75. That means, you need to find three numbers:
N = our common denominator.
x = what we will multiply 90 by.
y = what we will multiply 75 by.
Which gives us:
and:
The easiest common denominator can always be found by simply multiplying the two denominators that you have in your original fractions, like this:
and:
which gives us:
But these numbers are so big that they are a mess to work with. To find a smaller common denominator, we break our numbers into factors and see whether there are any duplicates:
so:
and:
so:
Aha! Both denominators have a factor of 3×5, so we can pull that out:
and:
Comparing these equations, can you see how to make them equal? Let x=5, and let y=2×3=6, which will give you:
Finally, going back to your original equation, you need to make equivalent fractions:
I think you can finish it from there.
how would you solve for n in this fraction problem
7 2/3 + n= 9 1/12
You have a number of options in approaching a problem like this. The standard approach is to subtract. If the big number is the sum of two smaller parts, and if you take away the part that you know, then what is left will be the other part.
An easier method for mental math is to “add up” — which means to start at the smaller number and see how much you have to add to get to the larger number. You don’t add the whole amount at once, but do it in easy chunks. How much would you add to get to 8? And then to 9? Then finally add the extra 1/12.
Or here is an algebra trick: You can multiply through by a common denominator to get rid of the fractions. First you would have to convert the mixed numbers, because you NEVER work with mixed numbers in algebra. Then multiply both sides of the equation by the common denominator. Finally, solve the resulting linear equation for your unknown.
I’m sorry, people, but I don’t want to do your homework for you. Try one of the forums listed on the Free Resources page. I will delete all future homework problem posts.
I just came across your website and found it very interesting. I have another method I came across in a teacher magazine that a sixth grade student had figured out. Add a whole amount to the fraction part on the top, e.g. 4/4 to the 1/4 in the original example resulting in 5/4.
Then add a whole amount to the whole number being subtracted, e.g. 1 + 2 in the original example resulting in 3. Then you find common denominators and subtract the fraction part. Next subtract the whole numbers. You don’t need to worry about borrowing this way. You could also find the common denominators first and then add the whole one to the fraction part as this might make it easier for some.
hey denise i find just using decimals easier than just using fractions, its pretty easy
Hi, Katerina!
Using decimals may be easier when you are working with a calculator, and that is fine for many practical uses of “real life” math. But I see two big problems with relying on decimals:
(1) Putting the numbers on a calculator does NOT help you understand them. Can you explain why 3/7 comes out as the decimal 0.428571429 (or whatever your calculator gives)?
(2) Decimals are not as accurate as fractions. The fraction 3/7 is very precise, but the decimal equivalent would go on forever. Therefore, we have to stop it somewhere and round it off, and then we have only an approximate answer.
I do not allow calculators in elementary or middle school math class. Nevertheless, sometimes decimals are easier (for instance, 0.5 compared to 1/2), and if a student can convert the fraction to a decimal and do the work that way — while still getting an accurate answer — I don’t mind.